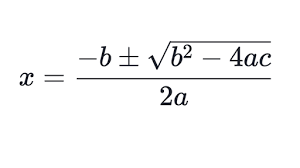Today I am going to be looking at a complicated word problem that an internet friend from Australia shared with me. (Apparently it was on ‘Reddit’ but I rarely look at that site)
Of course we need to use algebra to solve the problem, but forming the equations did take some thought.

The first equation doesn’t give to much difficulty. We let A’s current age be a, and B’s current age be b.
a = b + 15
Making the second equation
But what about the second sentence. That made my brain fry, just a little, when I first saw it. And my heading is a bit inaccurate, because I started to make not one extra equation, but two extras.
And I am going to use an extra letter, c
Three different Times
There are 3 different times in the question. There is the current time, and we already have an equation for now.
Then there the time when ‘A is as old as B will be’
So at that time B is ‘a’ years old . The age of A at that point is described by the rest of the sentence, and we haven’t decoded that. But we do know the age of A then is 4 times something, so I am going to say A = 4c
We know 4c = a + 15 : Because at this different point of time, A is still 15 years older than B
We now need to decode the last part.
When B was c years old, A = b/2 + 16 – sixteen years older than 1/2 of B’s current age
So using the 15 year old difference between the ages of A and B again
b/2 + 16 = c + 15
We can simplify this by taking 15 from both sides
c = b/2 + 1
I’m now going to substitute that expression for c into the earlier one
4(b/2 + 1) = a + 15
Simplify to give
2b + 4 = a + 15
so a = 2b – 11
We also know that a = b + 15
so 2b – 11 = b + 15, that gives us b = 26, so a = 41
Conclusion
There is nothing particularly special about this complicated word problem, other than it was convoluted and took an extra step of algebra for me to see a solution.
For more Maths word problems, see here

 This graph has been created by
This graph has been created by