The easiest way to learn constructions with compass and straight edge
I do find constructions with compass and straight edge is one topic that is easiest to tutor by watching videos together
I know how to do them, but when I have previously taught large groups , I have let others do the demonstration by using YouTube Videos!
In fact, I am going to do the same in this post!! But first let me explain some history, because constructions with a compass and straight edge have a place in ancient history. It was one of those things the Greeks of antiquity liked to do. In fact it was they who chose the rules.
So what exactly are these ‘Constructions’? Basically they are exercises in Geometry. There are a few of them but here we will look at three.
The constructions – What are they?
The first asks use to bisect a given line. Bisect means ‘divide into 2 equal parts. (Note all these drawings are example sketches. They have not been created by constriction. See the videos below on how to do those.)

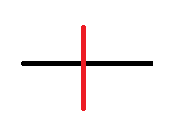
Then draw a perpendicular line at a chosen point on a line.
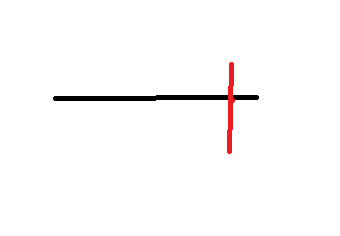
And then, Bisect an angle

You might be thinking – well that looks easy enough; just use my ruler and protractor. But that is because I haven’t shared the major catch; You can only use a pair of compasses and a straight edge. The rules say specifically a straight edge – You can use a ruler, for this BUT you have to ignore the markings! This is NOT a measuring exercise.
Why these restrictrictions? Were those ancient Greeks being just plain awkward? Well maybe, but also, they may not have had a protractor, but mostly this is an exercise in how things fit together.
This is the point where I hand over to other experts…..
Here are some more videos showing these constructions